LIEM synthesizes field measurements with a priori knowledge of ecosystem structure and physiological capabilities, to constrain material flows through an ecosystem.
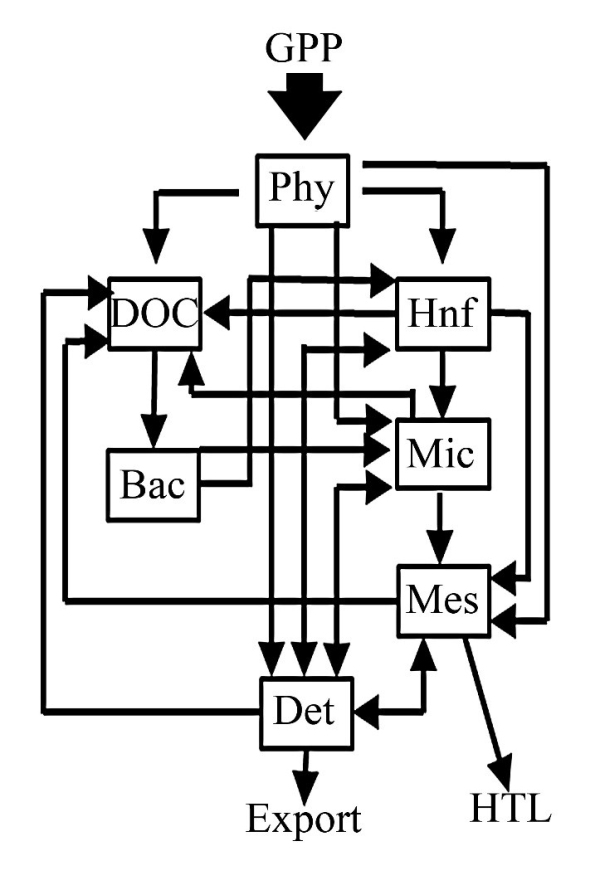
Simplified food-web structure used in LIEM, arrows in the figure are not proportional to flows. Input of material/energy to the system is GGP = gross primary production.
Compartments are: Phy = phytoplankton; Hnf = heterotrophic nanoflagellates; mic = microzooplankton; mes = Mesozooplankton; det = detritus; DOC = dissolved organic carbon; Bac = bacteria.
Loss terms include: Export = gravitational flux, and HTL = higher trophic levels. Respiration is included in the model but not in the figure for simplicity.
Model summary
LIEM is a powerful tool for synthesizing field measurements with a priori knowledge of ecosystem structure and physiological capabilities, to constrain material flows through an ecosystem (Vezina and Platt 1988; Van Oevelen et al. 2010). LIEM seeks to minimize the model-data mismatch with a series of measurements done during NIWA research voyages, while satisfying a series of mass balance and greater than/less than constraints. These codify known organismal constraints, such as gross growth efficiency (GGE) and respiration limits. To minimize food web uncertainty, a random walk Markov Chain Monte Carlo approach can be used to fully sample the solution space of the multi-dimensional LIEMs (Kones et al. 2009; Van Den Meersche et al. 2009).
References
Kones, J. K., K. Soetaert, D. Van Oevelen, and J. O. Owino. 2009. Are network indices robust indicators of food web functioning? A Monte Carlo approach. Ecol. Model. 220: 370-382, doi: 10.1016/j.ecolmodel.2008.10.012.
Stukel, M. R., M. R. Landry, M. D. Ohman, R. Goericke, T. Samo, and C. R. Benitez-Nelson. 2012. Do inverse ecosystem models accurately reconstruct plankton trophic flows? Comparing two solution methods using field data from the California Current. J. Mar. Syst. 91: 20-33, doi: 10.1016/j.jmarsys.2011.09.004.
Van Den Meersche, K., K. Soetaert, and D. Van Oevelen. 2009. xSample(): An R function for sampling linear inverse problems. Journal of Statistal Software, Code Snippets 30: 1-15.
Van Oevelen, D., K. Van Den Meersche, F. J. R. Meysman, K. Soetaert, J. J. Middelburg, and A. F. Vezina. 2010. Quantifying Food Web Flows Using Linear Inverse Models. Ecosystems 13: 32-45, doi: 10.1007/s10021-009-9297-6.
Vezina, A. F., and T. Platt. 1988. Food web dynamics in the ocean .1. Best-estimates of flow networks using inverse methods. Mar. Ecol. Prog. Ser. 42: 269-287.